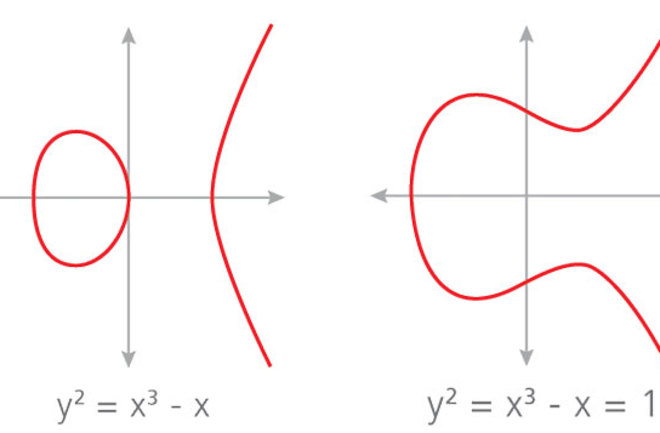
In high school, American students encounter a kind of wormhole connecting geometry and algebra: Geometric objects like circles and ellipses are linked to algebraic equations like y^2 = x^3 –x + 1. Students can visualize all the solutions to the equation as points in the circle — that is, each point in the curve represents a solution.
Make the equations slightly more complex, and instead of circles you get swooping loops and lines called elliptic curves. These curves have proven useful in creating cryptographic codes — and they have beguiled mathematicians with their complexity and beauty.
Each of those more complicated, curvy equations, however, has many solutions, and they can be wickedly hard to find. Mathematicians developed a trick for this: If they know a certain number of an equation’s solutions, they can use that wormhole between equation and curve to discover the rest of the equation’s solutions. The key is knowing exactly how many solutions are needed to unlock the rest.
A decade ago, a mathematician found one elliptic curve that required a whopping 28 solutions before its complete set of solutions could be known, way more than anyone expected. This created a renewed interest in the subject — might some curves require 50, or 500 or 5 billion solutions? Was there any limit at all?
It turns out the 28-solution monster from 2006 is a rarity. A new model published in February found that 21 solutions will almost always suffice, based on a statistical approach that simulates the behavior of elliptic curves. Yes, a few exceptional equations require 28 or more, but not many.
So far, the researchers have only produced a probabilistic argument, not a definitive proof. But already, the argument and its novel approach have led to a blossoming of new ideas in the field.